computerqert.blogg.se - Scalar equations of equilibrium 3d

The moment of inertia plays much the same role in rotational dynamics as mass does in linear dynamics, describing the relationship between angular momentum and angular velocity, torque and angular acceleration, and several other quantities. It is the inertia of a rotating body with respect to its rotation.

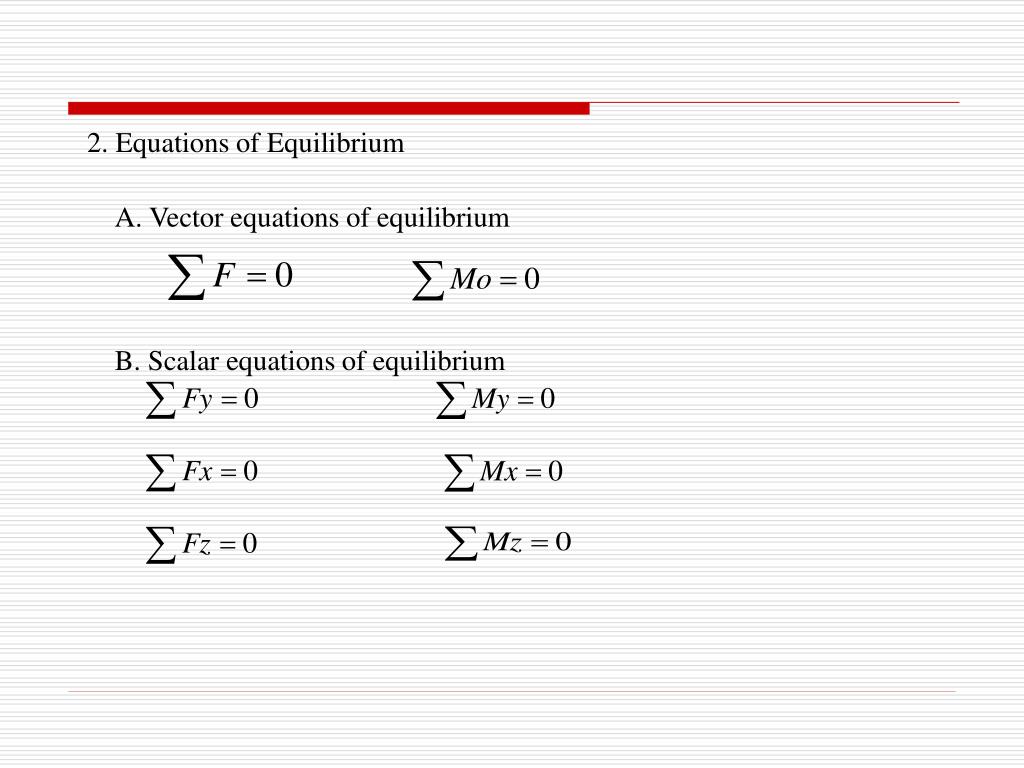
m²) is a measure of an object's resistance to changes to its rotation.

In classical mechanics, moment of inertia, also called mass moment, rotational inertia, polar moment of inertia of mass, or the angular mass, (SI units kg An engineering application of this concept is determining the tensions of up to three cables under load, for example the forces exerted on each cable of a hoist lifting an object or of guy wires restraining a hot air balloon to the ground. In a rectangular coordinate system the equilibrium equations can be represented by three scalar equations, where the sums of forces in all three directions are equal to zero. A particle is in equilibrium only if the resultant of all forces acting on the particle is equal to zero. The static equilibrium of a particle is an important concept in statics.

Varignon's theorem states that the moment of a force about any point is equal to the sum of the moments of the components of the force about the same point.

The application of Newton's second law to a system gives:į = m a. Statics is the branch of classical mechanics that is concerned with the analysis of force and torque (also called moment) acting on physical systems that do not experience an acceleration ( a=0), but rather, are in static equilibrium with their environment.